how to draw mohr's circle for 3d stress

Geometric civil engineering calculation technique

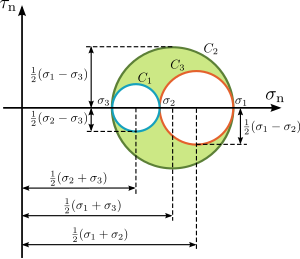
Figure one. Mohr's circles for a three-dimensional state of stress

Mohr'due south circle is a 2-dimensional graphical representation of the transformation law for the Cauchy stress tensor.

Mohr'southward circle is often used in calculations relating to mechanical engineering for materials' strength, geotechnical engineering for strength of soils, and structural technology for force of built structures. It is too used for calculating stresses in many planes by reducing them to vertical and horizontal components. These are called principal planes in which principal stresses are calculated; Mohr's circle can likewise be used to find the main planes and the principal stresses in a graphical representation, and is one of the easiest ways to do so.^[1]

After performing a stress analysis on a material body assumed as a continuum, the components of the Cauchy stress tensor at a particular material signal are known with respect to a coordinate organization. The Mohr circumvolve is then used to determine graphically the stress components acting on a rotated coordinate system, i.eastward., acting on a differently oriented plane passing through that point.

The abscissa and ordinate ( ${\displaystyle \sigma _{\mathrm {n} }}$ , ${\displaystyle \tau _{\mathrm {n} }}$ ) of each bespeak on the circumvolve are the magnitudes of the normal stress and shear stress components, respectively, acting on the rotated coordinate system. In other words, the circle is the locus of points that represent the state of stress on individual planes at all their orientations, where the axes stand for the master axes of the stress element.

19th-century German engineer Karl Culmann was the kickoff to excogitate a graphical representation for stresses while considering longitudinal and vertical stresses in horizontal beams during bending. His work inspired boyfriend German engineer Christian Otto Mohr (the circle's namesake), who extended information technology to both two- and three-dimensional stresses and developed a failure benchmark based on the stress circle.^[ii]

Alternative graphical methods for the representation of the stress state at a betoken include the Lamé's stress ellipsoid and Cauchy's stress quadric.

The Mohr circle tin be applied to any symmetric 2x2 tensor matrix, including the strain and moment of inertia tensors.

Motivation [edit]

Effigy ii. Stress in a loaded deformable cloth body assumed as a continuum.

Internal forces are produced betwixt the particles of a deformable object, assumed as a continuum, as a reaction to applied external forces, i.e., either surface forces or body forces. This reaction follows from Euler's laws of movement for a continuum, which are equivalent to Newton's laws of motility for a particle. A measure of the intensity of these internal forces is called stress. Considering the object is assumed as a continuum, these internal forces are distributed continuously inside the volume of the object.

In engineering, e.g., structural, mechanical, or geotechnical, the stress distribution within an object, for instance stresses in a rock mass around a tunnel, airplane wings, or building columns, is determined through a stress analysis. Computing the stress distribution implies the determination of stresses at every bespeak (material particle) in the object. According to Cauchy, the stress at any point in an object (Figure ii), assumed equally a continuum, is completely defined by the nine stress components ${\displaystyle \sigma _{ij}}$ of a second guild tensor of type (two,0) known as the Cauchy stress tensor, ${\displaystyle {\boldsymbol {\sigma }}}$ :

${\displaystyle {\boldsymbol {\sigma }}=\left[{\begin{matrix}\sigma _{11}&\sigma _{12}&\sigma _{13}\\\sigma _{21}&\sigma _{22}&\sigma _{23}\\\sigma _{31}&\sigma _{32}&\sigma _{33}\\\end{matrix}}\right]\equiv \left[{\begin{matrix}\sigma _{xx}&\sigma _{xy}&\sigma _{xz}\\\sigma _{yx}&\sigma _{yy}&\sigma _{yz}\\\sigma _{zx}&\sigma _{zy}&\sigma _{zz}\\\finish{matrix}}\right]\equiv \left[{\begin{matrix}\sigma _{ten}&\tau _{xy}&\tau _{xz}\\\tau _{yx}&\sigma _{y}&\tau _{yz}\\\tau _{zx}&\tau _{zy}&\sigma _{z}\\\end{matrix}}\right]}$

Figure iii. Stress transformation at a point in a continuum nether plane stress conditions.

After the stress distribution within the object has been determined with respect to a coordinate system ${\displaystyle (x,y)}$ , it may be necessary to calculate the components of the stress tensor at a particular material signal ${\displaystyle P}$ with respect to a rotated coordinate system ${\displaystyle (x',y')}$ , i.due east., the stresses acting on a plane with a dissimilar orientation passing through that point of interest —forming an angle with the coordinate system ${\displaystyle (x,y)}$ (Figure 3). For example, information technology is of interest to find the maximum normal stress and maximum shear stress, too every bit the orientation of the planes where they deed upon. To achieve this, information technology is necessary to perform a tensor transformation nether a rotation of the coordinate system. From the definition of tensor, the Cauchy stress tensor obeys the tensor transformation law. A graphical representation of this transformation police for the Cauchy stress tensor is the Mohr circumvolve for stress.

Mohr's circle for two-dimensional state of stress [edit]

Figure 4. Stress components at a aeroplane passing through a point in a continuum under airplane stress conditions.

In two dimensions, the stress tensor at a given material point ${\displaystyle P}$ with respect to any ii perpendicular directions is completely defined by merely three stress components. For the particular coordinate system ${\displaystyle (x,y)}$ these stress components are: the normal stresses ${\displaystyle \sigma _{x}}$ and ${\displaystyle \sigma _{y}}$ , and the shear stress ${\displaystyle \tau _{xy}}$ . From the balance of angular momentum, the symmetry of the Cauchy stress tensor can be demonstrated. This symmetry implies that ${\displaystyle \tau _{xy}=\tau _{yx}}$ . Thus, the Cauchy stress tensor can exist written equally:

${\displaystyle {\boldsymbol {\sigma }}=\left[{\begin{matrix}\sigma _{x}&\tau _{xy}&0\\\tau _{xy}&\sigma _{y}&0\\0&0&0\\\end{matrix}}\right]\equiv \left[{\brainstorm{matrix}\sigma _{x}&\tau _{xy}\\\tau _{xy}&\sigma _{y}\\\end{matrix}}\correct]}$

The objective is to use the Mohr circle to find the stress components ${\displaystyle \sigma _{\mathrm {n} }}$ and ${\displaystyle \tau _{\mathrm {north} }}$ on a rotated coordinate arrangement ${\displaystyle (x',y')}$ , i.east., on a differently oriented airplane passing through ${\displaystyle P}$ and perpendicular to the ${\displaystyle 10}$ - ${\displaystyle y}$ plane (Figure four). The rotated coordinate system ${\displaystyle (ten',y')}$ makes an angle ${\displaystyle \theta }$ with the original coordinate system ${\displaystyle (x,y)}$ .

Equation of the Mohr circle [edit]

To derive the equation of the Mohr circle for the two-dimensional cases of plane stress and plane strain, first consider a two-dimensional infinitesimal material element around a material signal ${\displaystyle P}$ (Figure 4), with a unit area in the management parallel to the ${\displaystyle y}$ - ${\displaystyle z}$ plane, i.e., perpendicular to the page or screen.

From equilibrium of forces on the infinitesimal chemical element, the magnitudes of the normal stress ${\displaystyle \sigma _{\mathrm {n} }}$ and the shear stress ${\displaystyle \tau _{\mathrm {due north} }}$ are given by:

${\displaystyle \sigma _{\mathrm {n} }={\frac {ane}{two}}(\sigma _{10}+\sigma _{y})+{\frac {1}{2}}(\sigma _{x}-\sigma _{y})\cos 2\theta +\tau _{xy}\sin 2\theta }$

${\displaystyle \tau _{\mathrm {northward} }=-{\frac {1}{2}}(\sigma _{10}-\sigma _{y})\sin two\theta +\tau _{xy}\cos two\theta }$

Derivation of Mohr's circle parametric equations - Equilibrium of forces

From equilibrium of forces in the direction of ${\displaystyle \sigma _{\mathrm {n} }}$ ( ${\displaystyle 10'}$ -axis) (Figure 4), and knowing that the area of the plane where ${\displaystyle \sigma _{\mathrm {n} }}$ acts is ${\displaystyle dA}$ , we have: ${\displaystyle \ {\begin{aligned}\sum F_{x'}&=\sigma _{\mathrm {n} }dA-\sigma _{x}dA\cos ^{2}\theta -\sigma _{y}dA\sin ^{2}\theta -\tau _{xy}dA\cos \theta \sin \theta -\tau _{xy}dA\sin \theta \cos \theta =0\\\sigma _{\mathrm {north} }&=\sigma _{x}\cos ^{2}\theta +\sigma _{y}\sin ^{two}\theta +2\tau _{xy}\sin \theta \cos \theta \\\end{aligned}}}$ However, knowing that ${\displaystyle \cos ^{two}\theta ={\frac {1+\cos 2\theta }{2}},\qquad \sin ^{2}\theta ={\frac {1-\cos two\theta }{ii}}\qquad {\text{and}}\qquad \sin two\theta =2\sin \theta \cos \theta }$ nosotros obtain ${\displaystyle \sigma _{\mathrm {n} }={\frac {i}{2}}(\sigma _{x}+\sigma _{y})+{\frac {1}{ii}}(\sigma _{x}-\sigma _{y})\cos 2\theta +\tau _{xy}\sin 2\theta }$ Now, from equilibrium of forces in the direction of ${\displaystyle \tau _{\mathrm {due north} }}$ ( ${\displaystyle y'}$ -axis) (Figure 4), and knowing that the area of the plane where ${\displaystyle \tau _{\mathrm {n} }}$ acts is ${\displaystyle dA}$ , nosotros have: ${\displaystyle \ {\begin{aligned}\sum F_{y'}&=\tau _{\mathrm {north} }dA+\sigma _{x}dA\cos \theta \sin \theta -\sigma _{y}dA\sin \theta \cos \theta -\tau _{xy}dA\cos ^{2}\theta +\tau _{xy}dA\sin ^{2}\theta =0\\\tau _{\mathrm {n} }&=-(\sigma _{x}-\sigma _{y})\sin \theta \cos \theta +\tau _{xy}\left(\cos ^{2}\theta -\sin ^{2}\theta \right)\\\end{aligned}}}$ However, knowing that ${\displaystyle \cos ^{2}\theta -\sin ^{2}\theta =\cos ii\theta \qquad {\text{and}}\qquad \sin ii\theta =ii\sin \theta \cos \theta }$ we obtain ${\displaystyle \tau _{\mathrm {n} }=-{\frac {1}{2}}(\sigma _{x}-\sigma _{y})\sin 2\theta +\tau _{xy}\cos 2\theta }$

Both equations can also be obtained by applying the tensor transformation law on the known Cauchy stress tensor, which is equivalent to performing the static equilibrium of forces in the direction of ${\displaystyle \sigma _{\mathrm {north} }}$ and ${\displaystyle \tau _{\mathrm {northward} }}$ .

Derivation of Mohr's circumvolve parametric equations - Tensor transformation

The stress tensor transformation law tin can exist stated every bit ${\displaystyle {\begin{aligned}{\boldsymbol {\sigma }}'&=\mathbf {A} {\boldsymbol {\sigma }}\mathbf {A} ^{T}\\\left[{\brainstorm{matrix}\sigma _{x'}&\tau _{x'y'}\\\tau _{y'x'}&\sigma _{y'}\\\cease{matrix}}\right]&=\left[{\begin{matrix}a_{x}&a_{xy}\\a_{yx}&a_{y}\\\finish{matrix}}\right]\left[{\brainstorm{matrix}\sigma _{ten}&\tau _{xy}\\\tau _{yx}&\sigma _{y}\\\cease{matrix}}\right]\left[{\begin{matrix}a_{x}&a_{yx}\\a_{xy}&a_{y}\\\end{matrix}}\right]\\&=\left[{\begin{matrix}\cos \theta &\sin \theta \\-\sin \theta &\cos \theta \\\end{matrix}}\right]\left[{\begin{matrix}\sigma _{x}&\tau _{xy}\\\tau _{yx}&\sigma _{y}\\\cease{matrix}}\right]\left[{\begin{matrix}\cos \theta &-\sin \theta \\\sin \theta &\cos \theta \\\end{matrix}}\right]\end{aligned}}}$ Expanding the right hand side, and knowing that ${\displaystyle \sigma _{x'}=\sigma _{\mathrm {northward} }}$ and ${\displaystyle \tau _{10'y'}=\tau _{\mathrm {n} }}$ , nosotros take: ${\displaystyle \sigma _{\mathrm {n} }=\sigma _{x}\cos ^{ii}\theta +\sigma _{y}\sin ^{two}\theta +2\tau _{xy}\sin \theta \cos \theta }$ However, knowing that ${\displaystyle \cos ^{two}\theta ={\frac {i+\cos 2\theta }{ii}},\qquad \sin ^{2}\theta ={\frac {ane-\cos 2\theta }{ii}}\qquad {\text{and}}\qquad \sin two\theta =2\sin \theta \cos \theta }$ we obtain ${\displaystyle \sigma _{\mathrm {due north} }={\frac {1}{2}}(\sigma _{x}+\sigma _{y})+{\frac {i}{two}}(\sigma _{x}-\sigma _{y})\cos two\theta +\tau _{xy}\sin 2\theta }$ ${\displaystyle \tau _{\mathrm {due north} }=-(\sigma _{x}-\sigma _{y})\sin \theta \cos \theta +\tau _{xy}\left(\cos ^{2}\theta -\sin ^{two}\theta \right)}$ Nevertheless, knowing that ${\displaystyle \cos ^{ii}\theta -\sin ^{two}\theta =\cos 2\theta \qquad {\text{and}}\qquad \sin ii\theta =2\sin \theta \cos \theta }$ we obtain ${\displaystyle \tau _{\mathrm {n} }=-{\frac {i}{2}}(\sigma _{x}-\sigma _{y})\sin two\theta +\tau _{xy}\cos 2\theta }$ It is not necessary at this moment to calculate the stress component ${\displaystyle \sigma _{y'}}$ acting on the airplane perpendicular to the plane of activeness of ${\displaystyle \sigma _{10'}}$ equally it is not required for deriving the equation for the Mohr circumvolve.

These two equations are the parametric equations of the Mohr circle. In these equations, ${\displaystyle ii\theta }$ is the parameter, and ${\displaystyle \sigma _{\mathrm {northward} }}$ and ${\displaystyle \tau _{\mathrm {n} }}$ are the coordinates. This means that past choosing a coordinate system with abscissa ${\displaystyle \sigma _{\mathrm {n} }}$ and ordinate ${\displaystyle \tau _{\mathrm {n} }}$ , giving values to the parameter ${\displaystyle \theta }$ will place the points obtained lying on a circle.

Eliminating the parameter ${\displaystyle 2\theta }$ from these parametric equations volition yield the not-parametric equation of the Mohr circle. This can be accomplished by rearranging the equations for ${\displaystyle \sigma _{\mathrm {northward} }}$ and ${\displaystyle \tau _{\mathrm {northward} }}$ , outset transposing the beginning term in the showtime equation and squaring both sides of each of the equations so adding them. Thus we take

${\displaystyle {\begin{aligned}\left[\sigma _{\mathrm {northward} }-{\tfrac {1}{ii}}(\sigma _{x}+\sigma _{y})\correct]^{two}+\tau _{\mathrm {north} }^{2}&=\left[{\tfrac {one}{2}}(\sigma _{x}-\sigma _{y})\right]^{2}+\tau _{xy}^{2}\\(\sigma _{\mathrm {n} }-\sigma _{\mathrm {avg} })^{ii}+\tau _{\mathrm {due north} }^{2}&=R^{2}\cease{aligned}}}$

where

${\displaystyle R={\sqrt {\left[{\tfrac {1}{ii}}(\sigma _{x}-\sigma _{y})\correct]^{2}+\tau _{xy}^{2}}}\quad {\text{and}}\quad \sigma _{\mathrm {avg} }={\tfrac {one}{ii}}(\sigma _{x}+\sigma _{y})}$

This is the equation of a circumvolve (the Mohr circle) of the form

${\displaystyle (x-a)^{2}+(y-b)^{ii}=r^{2}}$

with radius ${\displaystyle r=R}$ centered at a indicate with coordinates ${\displaystyle (a,b)=(\sigma _{\mathrm {avg} },0)}$ in the ${\displaystyle (\sigma _{\mathrm {n} },\tau _{\mathrm {n} })}$ coordinate system.

Sign conventions [edit]

There are two separate sets of sign conventions that need to be considered when using the Mohr Circumvolve: One sign convention for stress components in the "physical space", and another for stress components in the "Mohr-Circle-infinite". In improver, within each of the two set of sign conventions, the engineering mechanics (structural engineering and mechanical engineering) literature follows a different sign convention from the geomechanics literature. At that place is no standard sign convention, and the choice of a particular sign convention is influenced by convenience for calculation and interpretation for the particular problem in hand. A more detailed explanation of these sign conventions is presented below.

The previous derivation for the equation of the Mohr Circle using Figure 4 follows the engineering mechanics sign convention. The applied science mechanics sign convention will be used for this commodity.

Physical-space sign convention [edit]

From the convention of the Cauchy stress tensor (Effigy 3 and Figure iv), the first subscript in the stress components denotes the face on which the stress component acts, and the 2d subscript indicates the direction of the stress component. Thus ${\displaystyle \tau _{xy}}$ is the shear stress acting on the face with normal vector in the positive direction of the ${\displaystyle x}$ -centrality, and in the positive management of the ${\displaystyle y}$ -axis.

In the physical-space sign convention, positive normal stresses are outward to the plane of action (tension), and negative normal stresses are inward to the aeroplane of activeness (compression) (Figure 5).

In the concrete-space sign convention, positive shear stresses act on positive faces of the cloth element in the positive direction of an axis. Likewise, positive shear stresses act on negative faces of the fabric chemical element in the negative direction of an axis. A positive face has its normal vector in the positive direction of an axis, and a negative face has its normal vector in the negative direction of an axis. For instance, the shear stresses ${\displaystyle \tau _{xy}}$ and ${\displaystyle \tau _{yx}}$ are positive because they deed on positive faces, and they act every bit well in the positive direction of the ${\displaystyle y}$ -axis and the ${\displaystyle x}$ -centrality, respectively (Effigy iii). Similarly, the respective opposite shear stresses ${\displaystyle \tau _{xy}}$ and ${\displaystyle \tau _{yx}}$ acting in the negative faces have a negative sign because they human activity in the negative direction of the ${\displaystyle x}$ -centrality and ${\displaystyle y}$ -axis, respectively.

Mohr-circumvolve-infinite sign convention [edit]

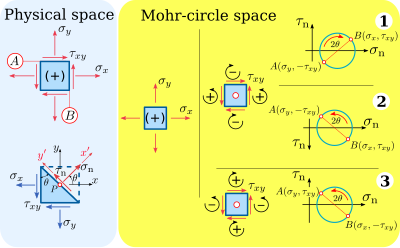
Figure v. Engineering mechanics sign convention for drawing the Mohr circle. This article follows sign-convention # iii, every bit shown.

In the Mohr-circle-space sign convention, normal stresses have the aforementioned sign as normal stresses in the physical-space sign convention: positive normal stresses act outward to the plane of action, and negative normal stresses human activity inwards to the plane of action.

Shear stresses, withal, have a different convention in the Mohr-circumvolve infinite compared to the convention in the physical space. In the Mohr-circle-space sign convention, positive shear stresses rotate the textile chemical element in the counterclockwise management, and negative shear stresses rotate the material in the clockwise direction. This manner, the shear stress component ${\displaystyle \tau _{xy}}$ is positive in the Mohr-circle space, and the shear stress component ${\displaystyle \tau _{yx}}$ is negative in the Mohr-circle space.

Two options exist for drawing the Mohr-circle space, which produce a mathematically correct Mohr circumvolve:

1. Positive shear stresses are plotted up (Figure 5, sign convention #ane)
2. Positive shear stresses are plotted down, i.e., the ${\displaystyle \tau _{\mathrm {northward} }}$ -axis is inverted (Figure v, sign convention #2).

Plotting positive shear stresses upwards makes the angle ${\displaystyle 2\theta }$ on the Mohr circle have a positive rotation clockwise, which is reverse to the concrete infinite convention. That is why some authors^[three] adopt plotting positive shear stresses down, which makes the angle ${\displaystyle 2\theta }$ on the Mohr circle have a positive rotation counterclockwise, similar to the physical space convention for shear stresses.

To overcome the "upshot" of having the shear stress axis downwards in the Mohr-circle infinite, there is an alternative sign convention where positive shear stresses are assumed to rotate the material element in the clockwise direction and negative shear stresses are causeless to rotate the cloth element in the counterclockwise management (Figure 5, option iii). This mode, positive shear stresses are plotted upward in the Mohr-circle space and the bending ${\displaystyle ii\theta }$ has a positive rotation counterclockwise in the Mohr-circle infinite. This alternative sign convention produces a circle that is identical to the sign convention #ii in Effigy v because a positive shear stress ${\displaystyle \tau _{\mathrm {n} }}$ is besides a counterclockwise shear stress, and both are plotted downward. Besides, a negative shear stress ${\displaystyle \tau _{\mathrm {n} }}$ is a clockwise shear stress, and both are plotted upwards.

This article follows the engineering science mechanics sign convention for the concrete space and the culling sign convention for the Mohr-circle space (sign convention #3 in Effigy 5)

Cartoon Mohr'due south circumvolve [edit]

Bold nosotros know the stress components ${\displaystyle \sigma _{x}}$ , ${\displaystyle \sigma _{y}}$ , and ${\displaystyle \tau _{xy}}$ at a indicate ${\displaystyle P}$ in the object under report, as shown in Figure iv, the following are the steps to construct the Mohr circle for the land of stresses at ${\displaystyle P}$ :

1. Draw the Cartesian coordinate organisation ${\displaystyle (\sigma _{\mathrm {northward} },\tau _{\mathrm {due north} })}$ with a horizontal ${\displaystyle \sigma _{\mathrm {due north} }}$ -centrality and a vertical ${\displaystyle \tau _{\mathrm {n} }}$ -axis.
2. Plot two points ${\displaystyle A(\sigma _{y},\tau _{xy})}$ and ${\displaystyle B(\sigma _{x},-\tau _{xy})}$ in the ${\displaystyle (\sigma _{\mathrm {northward} },\tau _{\mathrm {due north} })}$ space respective to the known stress components on both perpendicular planes ${\displaystyle A}$ and ${\displaystyle B}$ , respectively (Figure 4 and half-dozen), following the chosen sign convention.
3. Describe the bore of the circle by joining points ${\displaystyle A}$ and ${\displaystyle B}$ with a straight line ${\displaystyle {\overline {AB}}}$ .
4. Describe the Mohr Circumvolve. The centre ${\displaystyle O}$ of the circumvolve is the midpoint of the diameter line ${\displaystyle {\overline {AB}}}$ , which corresponds to the intersection of this line with the ${\displaystyle \sigma _{\mathrm {northward} }}$ centrality.

Finding principal normal stresses [edit]

Stress components on a second rotating chemical element. Example of how stress components vary on the faces (edges) of a rectangular chemical element as the angle of its orientation is varied. Chief stresses occur when the shear stresses simultaneously disappear from all faces. The orientation at which this occurs gives the principal directions. In this case, when the rectangle is horizontal, the stresses are given past ${\displaystyle \left[{\brainstorm{matrix}\sigma _{xx}&\tau _{xy}\\\tau _{yx}&\sigma _{yy}\finish{matrix}}\right]=\left[{\begin{matrix}-10&x\\ten&xv\end{matrix}}\correct].}$ The corresponding Mohr's circle representation is shown at the bottom.

The magnitude of the principal stresses are the abscissas of the points ${\displaystyle C}$ and ${\displaystyle E}$ (Effigy 6) where the circumvolve intersects the ${\displaystyle \sigma _{\mathrm {due north} }}$ -axis. The magnitude of the major principal stress ${\displaystyle \sigma _{1}}$ is e'er the greatest absolute value of the abscissa of any of these ii points. Too, the magnitude of the minor principal stress ${\displaystyle \sigma _{2}}$ is always the lowest accented value of the abscissa of these two points. As expected, the ordinates of these two points are nada, corresponding to the magnitude of the shear stress components on the principal planes. Alternatively, the values of the principal stresses can be found by

${\displaystyle \sigma _{one}=\sigma _{\max }=\sigma _{\text{avg}}+R}$

${\displaystyle \sigma _{2}=\sigma _{\min }=\sigma _{\text{avg}}-R}$

where the magnitude of the average normal stress ${\displaystyle \sigma _{\text{avg}}}$ is the abscissa of the centre ${\displaystyle O}$ , given by

${\displaystyle \sigma _{\text{avg}}={\tfrac {1}{2}}(\sigma _{10}+\sigma _{y})}$

and the length of the radius ${\displaystyle R}$ of the circle (based on the equation of a circumvolve passing through ii points), is given by

${\displaystyle R={\sqrt {\left[{\tfrac {1}{2}}(\sigma _{x}-\sigma _{y})\correct]^{two}+\tau _{xy}^{2}}}}$

Finding maximum and minimum shear stresses [edit]

The maximum and minimum shear stresses correspond to the ordinates of the highest and lowest points on the circumvolve, respectively. These points are located at the intersection of the circle with the vertical line passing through the heart of the circle, ${\displaystyle O}$ . Thus, the magnitude of the maximum and minimum shear stresses are equal to the value of the circle's radius ${\displaystyle R}$

${\displaystyle \tau _{\max ,\min }=\pm R}$

Finding stress components on an arbitrary plane [edit]

As mentioned before, after the two-dimensional stress assay has been performed we know the stress components ${\displaystyle \sigma _{x}}$ , ${\displaystyle \sigma _{y}}$ , and ${\displaystyle \tau _{xy}}$ at a cloth betoken ${\displaystyle P}$ . These stress components human activity in two perpendicular planes ${\displaystyle A}$ and ${\displaystyle B}$ passing through ${\displaystyle P}$ as shown in Figure 5 and vi. The Mohr circle is used to find the stress components ${\displaystyle \sigma _{\mathrm {north} }}$ and ${\displaystyle \tau _{\mathrm {n} }}$ , i.e., coordinates of any signal ${\displaystyle D}$ on the circle, interim on any other plane ${\displaystyle D}$ passing through ${\displaystyle P}$ making an bending ${\displaystyle \theta }$ with the plane ${\displaystyle B}$ . For this, two approaches can exist used: the double angle, and the Pole or origin of planes.

Double angle [edit]

Equally shown in Figure 6, to determine the stress components ${\displaystyle (\sigma _{\mathrm {n} },\tau _{\mathrm {north} })}$ acting on a plane ${\displaystyle D}$ at an angle ${\displaystyle \theta }$ counterclockwise to the airplane ${\displaystyle B}$ on which ${\displaystyle \sigma _{ten}}$ acts, we travel an angle ${\displaystyle 2\theta }$ in the same counterclockwise management around the circle from the known stress point ${\displaystyle B(\sigma _{x},-\tau _{xy})}$ to point ${\displaystyle D(\sigma _{\mathrm {n} },\tau _{\mathrm {n} })}$ , i.east., an angle ${\displaystyle 2\theta }$ between lines ${\displaystyle {\overline {OB}}}$ and ${\displaystyle {\overline {OD}}}$ in the Mohr circle.

The double angle approach relies on the fact that the bending ${\displaystyle \theta }$ between the normal vectors to any two concrete planes passing through ${\displaystyle P}$ (Effigy iv) is half the angle between two lines joining their corresponding stress points ${\displaystyle (\sigma _{\mathrm {northward} },\tau _{\mathrm {n} })}$ on the Mohr circle and the eye of the circle.

This double angle relation comes from the fact that the parametric equations for the Mohr circle are a function of ${\displaystyle 2\theta }$ . Information technology can also be seen that the planes ${\displaystyle A}$ and ${\displaystyle B}$ in the material chemical element effectually ${\displaystyle P}$ of Figure 5 are separated by an angle ${\displaystyle \theta =90^{\circ }}$ , which in the Mohr circle is represented past a ${\displaystyle 180^{\circ }}$ angle (double the angle).

Pole or origin of planes [edit]

Figure seven. Mohr'south circle for plane stress and plane strain atmospheric condition (Pole approach). Any straight line drawn from the pole will intersect the Mohr circumvolve at a point that represents the country of stress on a plane inclined at the same orientation (parallel) in space as that line.

The second approach involves the determination of a point on the Mohr circle chosen the pole or the origin of planes. Any straight line fatigued from the pole will intersect the Mohr circle at a point that represents the state of stress on a plane inclined at the same orientation (parallel) in space as that line. Therefore, knowing the stress components ${\displaystyle \sigma }$ and ${\displaystyle \tau }$ on any particular aeroplane, one can draw a line parallel to that plane through the item coordinates ${\displaystyle \sigma _{\mathrm {north} }}$ and ${\displaystyle \tau _{\mathrm {n} }}$ on the Mohr circle and find the pole equally the intersection of such line with the Mohr circumvolve. As an example, let's assume we have a state of stress with stress components ${\displaystyle \sigma _{x},\!}$ , ${\displaystyle \sigma _{y},\!}$ , and ${\displaystyle \tau _{xy},\!}$ , every bit shown on Figure 7. Beginning, we can depict a line from point ${\displaystyle B}$ parallel to the plane of activity of ${\displaystyle \sigma _{10}}$ , or, if nosotros choose otherwise, a line from indicate ${\displaystyle A}$ parallel to the airplane of action of ${\displaystyle \sigma _{y}}$ . The intersection of whatsoever of these two lines with the Mohr circle is the pole. One time the pole has been determined, to detect the state of stress on a airplane making an bending ${\displaystyle \theta }$ with the vertical, or in other words a plane having its normal vector forming an angle ${\displaystyle \theta }$ with the horizontal plane, then we can depict a line from the pole parallel to that airplane (Encounter Figure seven). The normal and shear stresses on that plane are then the coordinates of the point of intersection between the line and the Mohr circle.

Finding the orientation of the principal planes [edit]

The orientation of the planes where the maximum and minimum principal stresses act, also known every bit principal planes, tin exist determined by measuring in the Mohr circle the angles ∠BOC and ∠BOE, respectively, and taking half of each of those angles. Thus, the angle ∠BOC betwixt ${\displaystyle {\overline {OB}}}$ and ${\displaystyle {\overline {OC}}}$ is double the angle ${\displaystyle \theta _{p}}$ which the major principal aeroplane makes with plane ${\displaystyle B}$ .

Angles ${\displaystyle \theta _{p1}}$ and ${\displaystyle \theta _{p2}}$ can likewise be found from the following equation

${\displaystyle \tan 2\theta _{\mathrm {p} }={\frac {2\tau _{xy}}{\sigma _{y}-\sigma _{x}}}}$

This equation defines 2 values for ${\displaystyle \theta _{\mathrm {p} }}$ which are ${\displaystyle 90^{\circ }}$ autonomously (Figure). This equation can be derived straight from the geometry of the circle, or past making the parametric equation of the circumvolve for ${\displaystyle \tau _{\mathrm {n} }}$ equal to nil (the shear stress in the main planes is always zero).

Example [edit]

Assume a cloth element under a state of stress as shown in Figure 8 and Figure 9, with the aeroplane of one of its sides oriented ten° with respect to the horizontal plane. Using the Mohr circumvolve, find:

• The orientation of their planes of activity.
• The maximum shear stresses and orientation of their planes of action.
• The stress components on a horizontal airplane.

Check the answers using the stress transformation formulas or the stress transformation law.

Solution: Post-obit the engineering mechanics sign convention for the physical space (Figure 5), the stress components for the material element in this instance are:

${\displaystyle \sigma _{x'}=-ten{\textrm {MPa}}}$

${\displaystyle \sigma _{y'}=50{\textrm {MPa}}}$

${\displaystyle \tau _{x'y'}=40{\textrm {MPa}}}$ .

Post-obit the steps for drawing the Mohr circle for this particular state of stress, we kickoff draw a Cartesian coordinate organisation ${\displaystyle (\sigma _{\mathrm {n} },\tau _{\mathrm {n} })}$ with the ${\displaystyle \tau _{\mathrm {northward} }}$ -axis upward.

We and then plot two points A(fifty,twoscore) and B(-10,-xl), representing the state of stress at aeroplane A and B every bit show in both Figure 8 and Figure 9. These points follow the engineering science mechanics sign convention for the Mohr-circumvolve space (Figure 5), which assumes positive normals stresses outward from the fabric element, and positive shear stresses on each plane rotating the material chemical element clockwise. This way, the shear stress acting on plane B is negative and the shear stress acting on plane A is positive. The diameter of the circle is the line joining point A and B. The centre of the circle is the intersection of this line with the ${\displaystyle \sigma _{\mathrm {northward} }}$ -axis. Knowing both the location of the eye and length of the diameter, we are able to plot the Mohr circle for this particular state of stress.

The abscissas of both points E and C (Figure viii and Figure nine) intersecting the ${\displaystyle \sigma _{\mathrm {north} }}$ -axis are the magnitudes of the minimum and maximum normal stresses, respectively; the ordinates of both points E and C are the magnitudes of the shear stresses interim on both the small-scale and major principal planes, respectively, which is zilch for principal planes.

Even though the idea for using the Mohr circle is to graphically discover different stress components by really measuring the coordinates for unlike points on the circle, it is more convenient to ostend the results analytically. Thus, the radius and the abscissa of the eye of the circle are

${\displaystyle {\begin{aligned}R&={\sqrt {\left[{\tfrac {1}{2}}(\sigma _{x}-\sigma _{y})\right]^{two}+\tau _{xy}^{ii}}}\\&={\sqrt {\left[{\tfrac {1}{two}}(-10-50)\right]^{2}+twoscore^{2}}}\\&=50{\textrm {MPa}}\\\end{aligned}}}$

${\displaystyle {\begin{aligned}\sigma _{\mathrm {avg} }&={\tfrac {i}{2}}(\sigma _{x}+\sigma _{y})\\&={\tfrac {i}{two}}(-10+50)\\&=20{\textrm {MPa}}\\\end{aligned}}}$

and the principal stresses are

${\displaystyle {\brainstorm{aligned}\sigma _{1}&=\sigma _{\mathrm {avg} }+R\\&=70{\textrm {MPa}}\\\end{aligned}}}$

${\displaystyle {\begin{aligned}\sigma _{2}&=\sigma _{\mathrm {avg} }-R\\&=-thirty{\textrm {MPa}}\\\terminate{aligned}}}$

The coordinates for both points H and 1000 (Figure eight and Effigy 9) are the magnitudes of the minimum and maximum shear stresses, respectively; the abscissas for both points H and K are the magnitudes for the normal stresses interim on the same planes where the minimum and maximum shear stresses act, respectively. The magnitudes of the minimum and maximum shear stresses can be found analytically past

${\displaystyle \tau _{\max ,\min }=\pm R=\pm 50{\textrm {MPa}}}$

and the normal stresses acting on the same planes where the minimum and maximum shear stresses deed are equal to ${\displaystyle \sigma _{\mathrm {avg} }}$

We tin can cull to either use the double bending approach (Effigy 8) or the Pole approach (Figure 9) to find the orientation of the principal normal stresses and principal shear stresses.

Using the double bending approach we measure the angles ∠BOC and ∠BOE in the Mohr Circle (Figure 8) to find double the angle the major principal stress and the pocket-sized principal stress make with plane B in the concrete infinite. To obtain a more than authentic value for these angles, instead of manually measuring the angles, we can apply the belittling expression

${\displaystyle {\begin{aligned}2\theta _{\mathrm {p} }=\arctan {\frac {2\tau _{xy}}{\sigma _{x}-\sigma _{y}}}=\arctan {\frac {two*40}{(-10-50)}}=-\arctan {\frac {four}{3}}\end{aligned}}}$

One solution is: ${\displaystyle 2\theta _{p}=-53.13^{\circ }}$ . From inspection of Effigy eight, this value corresponds to the bending ∠BOE. Thus, the modest principal angle is

${\displaystyle \theta _{p2}=-26.565^{\circ }}$

Then, the major principal bending is

${\displaystyle {\begin{aligned}2\theta _{p1}&=180-53.13^{\circ }=126.87^{\circ }\\\theta _{p1}&=63.435^{\circ }\\\end{aligned}}}$

Remember that in this item example ${\displaystyle \theta _{p1}}$ and ${\displaystyle \theta _{p2}}$ are angles with respect to the plane of action of ${\displaystyle \sigma _{x'}}$ (oriented in the ${\displaystyle x'}$ -axis)and not angles with respect to the aeroplane of action of ${\displaystyle \sigma _{10}}$ (oriented in the ${\displaystyle x}$ -axis).

Using the Pole arroyo, we first localize the Pole or origin of planes. For this, nosotros draw through indicate A on the Mohr circle a line inclined ten° with the horizontal, or, in other words, a line parallel to plane A where ${\displaystyle \sigma _{y'}}$ acts. The Pole is where this line intersects the Mohr circle (Figure ix). To ostend the location of the Pole, we could draw a line through point B on the Mohr circle parallel to the plane B where ${\displaystyle \sigma _{x'}}$ acts. This line would as well intersect the Mohr circle at the Pole (Figure 9).

From the Pole, we draw lines to different points on the Mohr circle. The coordinates of the points where these lines intersect the Mohr circle indicate the stress components acting on a aeroplane in the physical space having the same inclination as the line. For example, the line from the Pole to point C in the circle has the same inclination as the plane in the physical space where ${\displaystyle \sigma _{1}}$ acts. This plane makes an bending of 63.435° with aeroplane B, both in the Mohr-circle space and in the physical space. In the aforementioned manner, lines are traced from the Pole to points E, D, F, G and H to find the stress components on planes with the same orientation.

Mohr'south circle for a general 3-dimensional state of stresses [edit]

Effigy x. Mohr'south circumvolve for a 3-dimensional state of stress

To construct the Mohr circle for a general three-dimensional case of stresses at a point, the values of the master stresses ${\displaystyle \left(\sigma _{1},\sigma _{2},\sigma _{three}\right)}$ and their primary directions ${\displaystyle \left(n_{1},n_{2},n_{three}\correct)}$ must be first evaluated.

Considering the principal axes as the coordinate organization, instead of the general ${\displaystyle x_{ane}}$ , ${\displaystyle x_{2}}$ , ${\displaystyle x_{3}}$ coordinate system, and bold that ${\displaystyle \sigma _{1}>\sigma _{2}>\sigma _{3}}$ , then the normal and shear components of the stress vector ${\displaystyle \mathbf {T} ^{(\mathbf {n} )}}$ , for a given plane with unit vector ${\displaystyle \mathbf {n} }$ , satisfy the following equations

${\displaystyle {\begin{aligned}\left(T^{(n)}\right)^{2}&=\sigma _{ij}\sigma _{ik}n_{j}n_{1000}\\\sigma _{\mathrm {n} }^{2}+\tau _{\mathrm {n} }^{two}&=\sigma _{one}^{two}n_{1}^{ii}+\sigma _{2}^{2}n_{2}^{2}+\sigma _{iii}^{2}n_{3}^{2}\end{aligned}}}$

${\displaystyle \sigma _{\mathrm {north} }=\sigma _{i}n_{1}^{2}+\sigma _{ii}n_{ii}^{two}+\sigma _{3}n_{3}^{2}.}$

Knowing that ${\displaystyle n_{i}n_{i}=n_{one}^{2}+n_{2}^{2}+n_{iii}^{two}=i}$ , nosotros can solve for ${\displaystyle n_{1}^{two}}$ , ${\displaystyle n_{2}^{2}}$ , ${\displaystyle n_{3}^{two}}$ , using the Gauss emptying method which yields

${\displaystyle {\begin{aligned}n_{1}^{two}&={\frac {\tau _{\mathrm {north} }^{2}+(\sigma _{\mathrm {n} }-\sigma _{2})(\sigma _{\mathrm {n} }-\sigma _{3})}{(\sigma _{1}-\sigma _{two})(\sigma _{one}-\sigma _{iii})}}\geq 0\\n_{2}^{2}&={\frac {\tau _{\mathrm {due north} }^{2}+(\sigma _{\mathrm {north} }-\sigma _{3})(\sigma _{\mathrm {due north} }-\sigma _{1})}{(\sigma _{2}-\sigma _{3})(\sigma _{2}-\sigma _{1})}}\geq 0\\n_{three}^{2}&={\frac {\tau _{\mathrm {due north} }^{2}+(\sigma _{\mathrm {n} }-\sigma _{1})(\sigma _{\mathrm {n} }-\sigma _{2})}{(\sigma _{iii}-\sigma _{1})(\sigma _{three}-\sigma _{2})}}\geq 0.\terminate{aligned}}}$

Since ${\displaystyle \sigma _{1}>\sigma _{2}>\sigma _{iii}}$ , and ${\displaystyle (n_{i})^{2}}$ is not-negative, the numerators from these equations satisfy

${\displaystyle \tau _{\mathrm {n} }^{2}+(\sigma _{\mathrm {north} }-\sigma _{2})(\sigma _{\mathrm {n} }-\sigma _{3})\geq 0}$ as the denominator ${\displaystyle \sigma _{1}-\sigma _{2}>0}$ and ${\displaystyle \sigma _{one}-\sigma _{iii}>0}$

${\displaystyle \tau _{\mathrm {due north} }^{ii}+(\sigma _{\mathrm {north} }-\sigma _{iii})(\sigma _{\mathrm {n} }-\sigma _{1})\leq 0}$ as the denominator ${\displaystyle \sigma _{2}-\sigma _{3}>0}$ and ${\displaystyle \sigma _{2}-\sigma _{1}<0}$

${\displaystyle \tau _{\mathrm {north} }^{2}+(\sigma _{\mathrm {n} }-\sigma _{1})(\sigma _{\mathrm {north} }-\sigma _{2})\geq 0}$ as the denominator ${\displaystyle \sigma _{iii}-\sigma _{1}<0}$ and ${\displaystyle \sigma _{3}-\sigma _{two}<0.}$

These expressions tin be rewritten as

${\displaystyle {\brainstorm{aligned}\tau _{\mathrm {n} }^{2}+\left[\sigma _{\mathrm {north} }-{\tfrac {1}{two}}(\sigma _{two}+\sigma _{3})\right]^{two}\geq \left({\tfrac {1}{2}}(\sigma _{ii}-\sigma _{3})\right)^{2}\\\tau _{\mathrm {due north} }^{2}+\left[\sigma _{\mathrm {n} }-{\tfrac {1}{2}}(\sigma _{ane}+\sigma _{3})\right]^{2}\leq \left({\tfrac {1}{2}}(\sigma _{ane}-\sigma _{iii})\right)^{2}\\\tau _{\mathrm {n} }^{2}+\left[\sigma _{\mathrm {n} }-{\tfrac {1}{two}}(\sigma _{1}+\sigma _{2})\right]^{2}\geq \left({\tfrac {1}{two}}(\sigma _{1}-\sigma _{2})\right)^{ii}\\\end{aligned}}}$

which are the equations of the three Mohr'due south circles for stress ${\displaystyle C_{one}}$ , ${\displaystyle C_{ii}}$ , and ${\displaystyle C_{3}}$ , with radii ${\displaystyle R_{1}={\tfrac {one}{2}}(\sigma _{two}-\sigma _{3})}$ , ${\displaystyle R_{2}={\tfrac {1}{two}}(\sigma _{ane}-\sigma _{iii})}$ , and ${\displaystyle R_{3}={\tfrac {1}{ii}}(\sigma _{1}-\sigma _{two})}$ , and their centres with coordinates ${\displaystyle \left[{\tfrac {one}{2}}(\sigma _{ii}+\sigma _{iii}),0\correct]}$ , ${\displaystyle \left[{\tfrac {1}{2}}(\sigma _{i}+\sigma _{3}),0\right]}$ , ${\displaystyle \left[{\tfrac {1}{2}}(\sigma _{1}+\sigma _{two}),0\right]}$ , respectively.

These equations for the Mohr circles evidence that all admissible stress points ${\displaystyle (\sigma _{\mathrm {north} },\tau _{\mathrm {northward} })}$ lie on these circles or within the shaded expanse enclosed past them (see Figure 10). Stress points ${\displaystyle (\sigma _{\mathrm {n} },\tau _{\mathrm {northward} })}$ satisfying the equation for circle ${\displaystyle C_{1}}$ prevarication on, or exterior circle ${\displaystyle C_{ane}}$ . Stress points ${\displaystyle (\sigma _{\mathrm {northward} },\tau _{\mathrm {n} })}$ satisfying the equation for circle ${\displaystyle C_{two}}$ lie on, or inside circle ${\displaystyle C_{2}}$ . And finally, stress points ${\displaystyle (\sigma _{\mathrm {n} },\tau _{\mathrm {due north} })}$ satisfying the equation for circle ${\displaystyle C_{3}}$ lie on, or outside circle ${\displaystyle C_{3}}$ .

See as well [edit]

• Critical plane assay

References [edit]

1. ^ "Principal stress and principal aeroplane". www.engineeringapps.internet . Retrieved 2019-12-25 .
2. ^ Parry, Richard Hawley Greyness (2004). Mohr circles, stress paths and geotechnics (2 ed.). Taylor & Francis. pp. 1–thirty. ISBN0-415-27297-i.
3. ^ Gere, James M. (2013). Mechanics of Materials. Goodno, Barry J. (8th ed.). Stamford, CT: Cengage Learning. ISBN9781111577735.

Bibliography [edit]

• Beer, Ferdinand Pierre; Elwood Russell Johnston; John T. DeWolf (1992). Mechanics of Materials . McGraw-Loma Professional. ISBN0-07-112939-ane.
• Brady, B.H.G.; Eastward.T. Brown (1993). Rock Mechanics For Cloak-and-dagger Mining (Tertiary ed.). Kluwer Academic Publisher. pp. 17–29. ISBN0-412-47550-two.
• Davis, R. O.; Selvadurai. A. P. Southward. (1996). Elasticity and geomechanics. Cambridge University Press. pp. 16–26. ISBN0-521-49827-9.
• Holtz, Robert D.; Kovacs, William D. (1981). An introduction to geotechnical engineering. Prentice-Hall civil engineering and applied science mechanics series. Prentice-Hall. ISBN0-13-484394-0.
• Jaeger, John Conrad; Cook, N.G.W.; Zimmerman, R.Due west. (2007). Fundamentals of rock mechanics (Fourth ed.). Wiley-Blackwell. pp. 9–41. ISBN978-0-632-05759-7.
• Jumikis, Alfreds R. (1969). Theoretical soil mechanics: with practical applications to soil mechanics and foundation engineering. Van Nostrand Reinhold Co. ISBN0-442-04199-3.
• Parry, Richard Hawley Grey (2004). Mohr circles, stress paths and geotechnics (2 ed.). Taylor & Francis. pp. i–xxx. ISBN0-415-27297-1.
• Timoshenko, Stephen P.; James Norman Goodier (1970). Theory of Elasticity (Third ed.). McGraw-Hill International Editions. ISBN0-07-085805-5.
• Timoshenko, Stephen P. (1983). History of strength of materials: with a brief account of the history of theory of elasticity and theory of structures. Dover Books on Physics. Dover Publications. ISBN0-486-61187-6.

External links [edit]

• Mohr'southward Circle and more circles by Rebecca Brannon
• DoITPoMS Didactics and Learning Package- "Stress Analysis and Mohr'due south Circle"

collierlittes1943.blogspot.com

Source: https://en.wikipedia.org/wiki/Mohr%27s_circle

Share this post